Valparaiso's dynamics working days: Groups and low dimensional dynamics

Introducción

The two-days conference  “Valparaiso’s dynamics working day: groups and low dimensional dynamics”  which will take place at Valparaiso, Chile, on October 15 -16th, 2015.

The idea is to gather researchers, postdocs and students interested on topics related to groups, groups action, low dimensional dynamic, entropy etc.  

Please help us to popularize the event by forwarding this information to interested students and colleagues at your institution. 

We invite you to participate in this workshop. If you want to give a talk for 45 minutes, please send a title and a summary.

A poster session will be available for those graduate students requiring support of their institutions to participate in this meeting.

Organizers

JAIRO BOCHI (PUC Chile)
email: jairo.bochi@mat.puc.cl
phone: (+56 02) 2354 1025

EDUARDO JORQUERA (PUCV)
email: eduardo.jorquera@pucv.cl
phone: (+56 32) 227 4039

CRISTOBAL RIVAS (USACH)
email: cristobal.rivas@usach.cl
phone:  (+56 2) 2718 1984

CARLOS H. VASQUEZ (PUCV)
email: carlos.vasquez@pucv.cl
phone: (+56 32) 227 4011

Confirmed speakers

Daniel Smania (USP-Sao Carlos, Brasil)

Juliana Xavier (U. de la República, Uruguay)
Juan Alonso (U. de la República, Uruguay)
Maria Isabel Cortez (Usach)
Maryam Hosseini (Usach- CMM)
Martin Sambarino (U. de la República, Uruguay)
Mario Ponce (PUC Chile )
Michele Triestino (PUC Rio)
Radu Saghin (PUCV Chile)
Ruben Hidalgo (Ufro, Chile)
Vincent Delecroix (U. Bordeaux, Francia)

Program

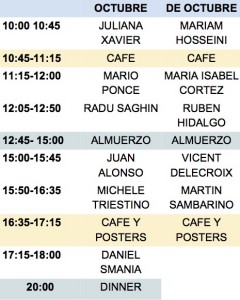
Talks

Growth rate of periodic points for self maps of the annulus.

Juliana Xavier

Universidad de la República, Uruguay

Consider a continuous self map of the annulus with degree $d>1$. It is proved that the number of Nielsen classes of periodic points is maximum possible whenever $f$ has a completely invariant essential continuum.

On the dynamics of 2-dimensional cylindrical vortices.

Mario Ponce

Pontificia Universidad Católica de Chile

A cylindrical vortex is a skew product dynamics with fiber map equal to an isometry. We study cylindrical vortices over minimal dynamics, and establish  results about  reducibility (conjugacy to rotation fiber maps), transitivity, minimality, quasi-reducibility, etc. Many different elementary techniques are used and important questions arise naturally from these apparently simple dynamical systems. These results are contained in works in collaboration with D.Coronel and A. navas.

Partially hyperbolic diffeomorphisms with one-dimensional center

Radu Saghin

Pontificia Universidad Católica de Valparaíso, Chile

The partially hyperbolic diffeomorphisms with the center bundle of dimension one are very close to the uniformly hyperbolic systems. The later ones are fairly well understood, and also a lot is known about dynamics in dimension one. Thus, one can hope to use various tools from uniform hyperbolicity and one-dimensional dynamics in order to study partially hyperbolic diffeomorphisms with one-dimensional center. I will present various results and several conjectures in this area.Groups of circle homeomorphisms with invariant laminations.

Groups of circle homeomorphisms with invariant laminations.

Juan Alonso

Universidad de la República, Uruguay

Together with H. Baik and E. Samperton, we study subgroups of Homeo+(S^1) according to the laminations they preserve. Our most specific goal is to characterize the main examples that arise from hyperbolic geometry of surfaces and 3-manifolds.

I will give a brief overview of this topic. Then I will focus on showing the Tits alternative for groups with two transverse invariant laminations (with suitable conditions).

About the ends of groups of circle diffeomorphisms, their dynamics and the algebraic structure

Michele Triestino

Puc-Rio, Brazil

The reason for studying groups of circle diffeomorphisms is mainly twofold: on the one hand it is the connection to the dynamics of codimension one foliations, on the other hand they represent somehow the smallest infinite dimensional Lie groups. It is natural to believe that the dynamical behaviour and the algebraic structure of such a group influence each other. For example, Denjoy’s theorem says that a virtually cyclic group can’t act with a minimal Cantor set. Another very interesting example comes from the theorems of Duminy and Ghys: a group of analytic diffeomorphisms with an invariant Cantor set is virtually free. In this talk, I’ll explain how we can consider that the groups that acts with an invariant Cantor set are examples of subgroups of “lattices” in the group of all circle diffeomorphisms. This constitutes an important step in our work towards the “classification” of groups of circle diffeomorphisms, which is an ongoing project with Sébastien Alvaréz (IMPA, RJ), Pablo Barrientos, Artem Raibekas (UFF, RJ), Dmitry Filimonov (HSE, Moscow), Victor Kleptsyn (CNRS & IRMAR, Rennes), Dominique Malicet (UERJ, RJ), Carlos Meniño (UFRJ, RJ) e Andrés Navas (USACH, Santiago de Chile).

Linear response: recents results

Daniel Smania

ICMC USP Sao Carlos, Brazil

We are going do discuss recents results on the differentiability of SBR measure with respect to the parameter in  families of piecewise expanding unimodal maps. In particular we are going to talk about results with Amanda de Lima  on the non differentiability of the SBR measure in transversal families of piecewise expanding unimodal maps.

On the Spectrum of Cantor Minimal Systems

Mariam Haseinni

Universidad de Santiago de Chile

I will talk about some recent results about the continuous eigenvalues of Cantor minimal systems. Some necessary and sufficient conditions for existence of such eigenvalues will be discussed.

Invariant measures and orbit equivalence classes for some group actions on the Cantor set

María Isabel Cortés

Universidad de Santiago de Chile

We show that for an amenable residually finite group, any possible set of invariant probability measure set is realizable among its minimal actions on the Cantor set. We discuss the possibility to extend this result to any (countable) amenable group. Joint work with Samuel Petite.

Funciones Racionales de Signatura Impar

Ruben Hidalgo

Universidad de la Frontera – Universidad Técnica Federico Santa María, Chile

Cada función racional $R in {mathbb C}(z)$, de grado $d$, tiene asociado un invariante algebraico por conjugación por transformaciones de Möbius; su cuerpo de moduli ${mathcal M}_{R}$. Este se puede ver como la intersección de todos los cuerpos de definición de $R$. El cuerpo de moduli es un cuerpo de definición si $d leq 1$. Cuando $d geq 2$, J. Silverman probó que esto también es cierto cuando $d$ es par o cuando$R$ es conjugado (por una transformación de Möbius) a un  polinomio. Cuando $d geq 3$ es impar, esto puede fallar; ejemplos fueron construidos por Silverman. El autor ha observado que toda función racional que no se define sobre su cuerpo de moduli puede ser definido sobre una cierta extensión de grado dos de este.

En esta charla introduciré el  concepto de “signatura impar” para una función racional;  esta propiedad es una condiciónsuficiente para que la función  racional se pueda definir sobre su cuerpo de moduli. Las funciones racionales de grado par y aquellas que son conjugadas a polinomios son ejemplos de funciones de signatura impar. Como una aplicación de esto, estudiaremos las funciones racionales de König de cualquier orden asociadas a polinomios (caso particular de estas funciones racionales son las provenientes del método de Newton). La noción de signatura impar para una función racional es una adaptación del concepto de signatura impar de orbifolds de género cero y que ha sido utilizado para problemas similares para el caso de curvas algebraicas.

Recurrencia de mapas del intervalo que preservan la medida de Lebesgue.

Vicent Delecroix

U. Bordeaux, France

Trabajo en conjunto con Michael Boshernitzan. Para una rotacíon $R: x -> x+a (mod 1)$ se conece de un resultado de Andrey Markoff (1879) que la límit inferior de $n$ $dist(R^n x, x)$ es menor que $1/sqrt{5}$. Probaremos que esta relación es valida por todos los mapas del intervalo $[0,1]$ que preservan la medida de Lebesgue.

Attracting circloids and entropy.

Martin Sambarino

U. de la República, Uruguay

We consider a map on the annulus having an attracting circloid and such that the rotation set on this circloid is nontrivial. We prove that it must have positive topological entropy. We further analize if the entropy has any relation with the size of the rotation set.

Posters

Restricted body problems in constant curvature surfaces

Jaime Andrade

U. Bio Bio, Chile

We derive the equations of motion of $N$ bodies with masses $m_1, …, m_N$  moving on a surface of constant curvature. In particular, we consider a restricted circular 3 body problem defined in the surfaces $S^2$ and $H^2$.

Classification of global phase portraits of Hamiltonian systems with rational potencial

 Yohanna Martinez

U. Bio Bio, Chile

We study the global dynamics of the Hamiltonian systems $latex dot x= H_{y}, dot y= -H_{x}$, where the Hamiltonian function $H$ has the particular form $H(x, y)=y^2/2+P(x)/Q(x)$, i.e., is the sum of the kinetic and rational potential energy. Firstly, we provide the normal forms by suitable $mu$-symplectic change of variables. Then, the global topological classification of the phase portraits of these systems having canonical forms in the Poincar’e disk are studied in the case where $mbox{degree}(P)=0,1,2$ and $mbox{degree}(Q)=0,1, 2$ as a function of the parameters that define each polynomial. We use blow-up technique for finite equilibrium points and the Poincar’e compactification for the infinite equilibrium points.

Topological entropy for impulsive semiflows

Nelda Jaque

U. Católica del Norte, Chile

In A variational principle for impulsive semiflows, by Alves, J., Carvalho, M. and Vasquez, C.,  a notion of topological entropy is given for some kind impulsive systems using the concept admissible sequence. In this work we give a little variation of the original definition of topological entropy though spanning and separated sets for continuous semiflows, this definition can be applied in the context of impulsive semiflows.

Participants

Adoreé Alvarado (USACH, Chile)

Andrés Navas (USACH, Chile)

Carlos Vásquez (PUCV, Chile)

Cristobal Rivas (USACH, Chile)

Daniel Smania (USP-Sao Carlos, Brasil)

Eder Contreras (U.Chile, Chile)

Eduardo Oregón (PUC, Chile)

Eduardo Jorquera (PUCV, Chile)

Enzo Fuentes (PUCV, Chile)

Felipe Pérez (PUC, Chile)

Ferran Valdez (UNAM, Mexico)

Francisco Valenzuela (PUCV, Chile)

Godofredo Iommi (PUC, Chile)

Jaime Andrade (U. Bio Bio, Chile)

Juan Alonso (U. de la República, Uruguay)

Juliana Xavier (U. de la República, Uruguay)

Maria Isabel Cortez (Usach)

Maria José Pacífico (UFRJ, Brazil)

Mario Ponce (PUC Chile )

Martin Sambarino (U. de la República, Uruguay)

Maryam Hosseini (Usach- CMM, Chile)

Matthieu Calvez (USACH, Chile)

Michele Triestino (PUC Rio, Brazil)

Nelda Jaque (UCN, Chile)

Radu Saghin (PUCV Chile)

Rosa Cisterna (USACH, Chile)

Ruben Hidalgo (Ufro, Chile)

Siming Tu (U. Chile)

Vincent Delecroix (U. Bordeaux, France)

Yohanna Martinez (U. Bio Bio, Chile)

Registration

Valparaíso

 The city-port of Valparaíso is a UNESCO World Heritage Site, located 111.8 km west of Santiago.  It is part of the Greater Valparaíso urban area (803,683 inhabitants) which also includes the neighbouring towns of Viña del Mar, Concón, Quilpué and Villa Alemana.

The nearest airport is Santiago International Airport (SCL).

Nicknamed “The Jewel of the Pacific”, Valparaíso was declared a world heritage site based upon its improvised urban design and unique architecture in 2003. Built upon dozens of steep hillsides overlooking the Pacific Ocean, Valparaíso boasts a labyrinth of streets and cobblestone alleyways, embodying a rich architectural and cultural legacy.

During Valparaíso’s golden age (1848–1914), the city received large numbers of immigrants, primarily from Europe. The immigrant communities left a unique imprint on the city’s noteworthy architecture. Each community built its own churches and schools, while many also founded other noteworthy cultural and economic institutions. The largest immigrant communities came from Britain, Germany, and Italy, each developing their own hillside neighbourhood, preserved today as National Historic Districts or “Zonas Típicas.”

Nightlife activities in Valparaíso are claimed to be among the best in the country^. Sailors and students alike favour the harbour sector due to the various traditional bars and nightclubs. A vivid guide to Valparaíso can be found in the novels of Cayetano Brule, the private detective who lives in a Victorian house, in the picturesque Paseo Gervasoni in Cerro Concepción.

Valparaíso is considered the graffiti capital of Chile. Amazing murals created by talented artists can be viewed on the streets, alleyways and stairways all over Valparaíso.

You can find more information about tours and activities here.

(Text taken and adapted from Wikipedia)

Sponsors

Instituto de Matemáticas PUCV

CONICYT

Center of Dynamical Systems and Related Fields